A Quick Lesson on Dividing Fractions

Objectives:

• Understand the concept of dividing fractions.

• Learn the steps to divide fractions using the "Keep, Change, Flip" method.

• Solve practice problems to reinforce the concept.

Introduction to Dividing Fractions

Hey there! Today we're going to tackle a topic that some find tricky: dividing fractions. But don’t worry; we’re going to make it as easy as pie.

The Concept of Dividing Fractions

Think about what dividing really means: When you divide, you’re figuring out how many times one number fits into another.

For example, if you have 8 cookies and want to share them equally among 4 friends, you'd divide 8 by 4, and each friend would get 2 cookies.

Now, let's say we want to divide fractions: Dividing fractions can feel a bit different, but with the right steps, it will be a piece of cake!

Step-by-Step Method: Keep, Change, Flip

The method we'll use to divide fractions is called "Keep, Change, Flip."

1. Keep the first fraction the same.

2. Change the division sign (÷) to a multiplication sign (×).

3. Flip the second fraction (take the reciprocal).

Example 1: Dividing Simple Fractions

Let’s look at an example!

Problem: ( 2/3 ÷ 4/5 )

Step 1: Keep.We keep the first fraction as it is: ( 2/3 ÷ 4/5 ).

Step 2: Change.We change the division to multiplication:Now we have ( 2/3 × ).

Step 3: Flip. Now we flip the second fraction (4/5 to get ( 5/4 ).

now our problem's like this:(2/3 × 5/4 ).

Step 4: Multiply. Now we multiply the numerators and the denominators:

Numerators: ( 2 × 5 = 10 )

Denominators: ( 3 × 4 = 12 )

So we get:( 10/12 ).

Step 5: Simplify (if possible).We can simplify (10/12 ) by dividing both the numerator and the denominator by their greatest common factor, which is 2

: ( 10/2 x 12/2 =5/6 ).

So:Final Answer: ( 2/3÷4/5 =5/6 ).

Example 2: Dividing Larger Fractions

Let’s do another example!

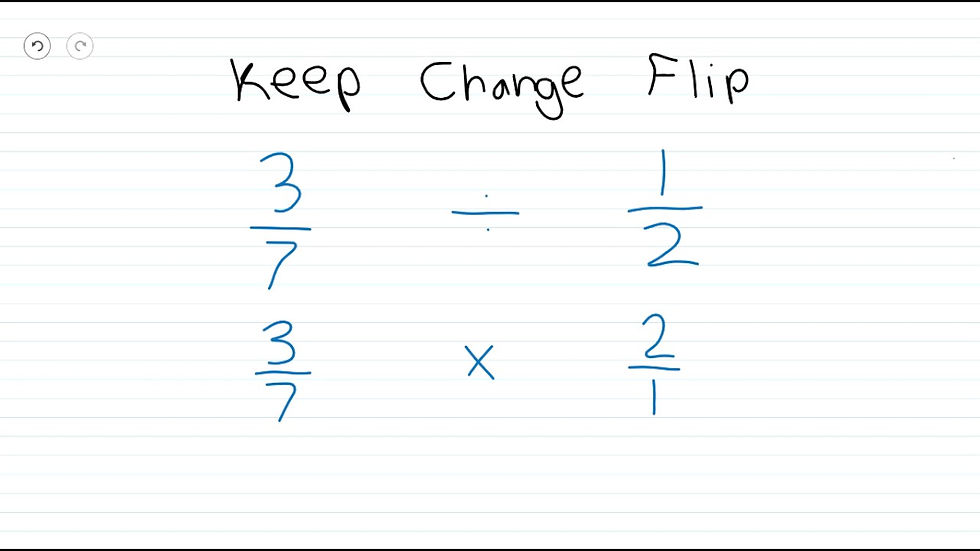
Problem: ( 3/4 ÷ 1/2 )

Step 1: Keep.Keep the first fraction: (3/4 ).

Step 2: Change.Change the division sign to multiplication:( 3/4 × ).

Step 3: Flip.Flip the second fraction ( 1/2 to get ( 2/1 ).

Now we have:( 3/4 × 2/1 ).

Step 4: Multiply.Multiply the numerators and denominators:

Numerators: ( 3 × 2 = 6 )

Denominators: ( 4 × 1 = 4 )

So we get:( 6/4 ).

Step 5: Simplify.We can simplify (6/4 ) by dividing both the numerator and the denominator by their greatest common factor, which is 2: (6/2 x 4/2 = 3/2 ).

So:Final Answer: ( 3/4 ÷1/2 = 3/2 ) or ( 1 , 1/2 ).

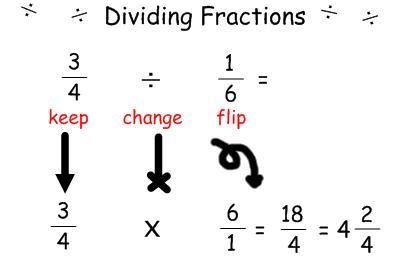
Practice Problems

Now it's your turn! Try these on your own:

1. ( 5/6 ÷ 2/3 )

2. ( 1/3} ÷ 1/6 )

3. ( 7/8} ÷ 1/4 )

Feel free to use the "Keep, Change, Flip" method as you work through these problems.

Great job today! Dividing fractions might seem complex, but with the “Keep, Change, Flip” method, it becomes pretty straightforward. Remember, practicing will help you get even better. Keep practicing, and soon dividing fractions will be as easy as pie!

Answers to Practice Problems

1. (5/6 ÷2/3 = 5/4 ) or ( 1,1/4 )

2. (1/3 ÷ 1/6 = 2 )

3. ( 7/8 ÷1/4 =7/25 ) or ( 3 ,1/2} )

Happy learning!